A Class of Models with the Potential to Represent Fundamental Physics
  1. Introduction
  2. Basic Form of Models
  3. Typical Behaviors
  4. Limiting Behavior and Emergent Geometry
  5. The Updating Process for String Substitution Systems
  6. The Updating Process in Our Models
  7. Equivalence and Computation in Our Models
  8. Potential Relation to Physics
  9. Additional Material
  10. References
  11. Index
Download PDf download pdf
ARXIV ARXIV
peer-review peer review
technical introduction Limiting Behavior and Emergent Geometry

4.3 Geometry from Subdivision

The grids and surfaces that we saw above were all produced by rules that end up executing a laborious “knitting” process in which they add just a single relation at each step. But it is also possible to generate recognizable geometric forms more quickly—in effect by a process of repeated subdivision.

Consider the 2312 4342 rule:

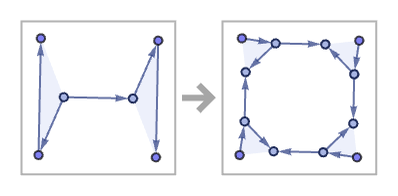
At each step, this rule doubles the number of relations—and quickly produces a structure with a definite emergent geometrical form:

After 10 steps the rule has generated 2560 relations, in the following structure:

Visualized in 3D, this becomes:

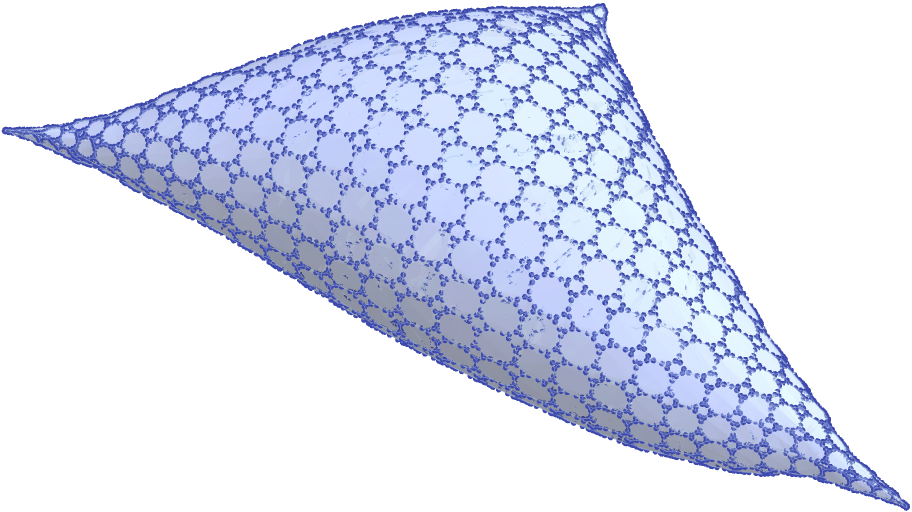
Once again, this corresponds to a smooth surface, but with 3 cusps. The surface is defined not by a simple triangular grid, but instead by an octagon-square (“truncated square”) tiling—that in this case becomes twice as fine at every step.

Changing the initial conditions can give a somewhat different structure:

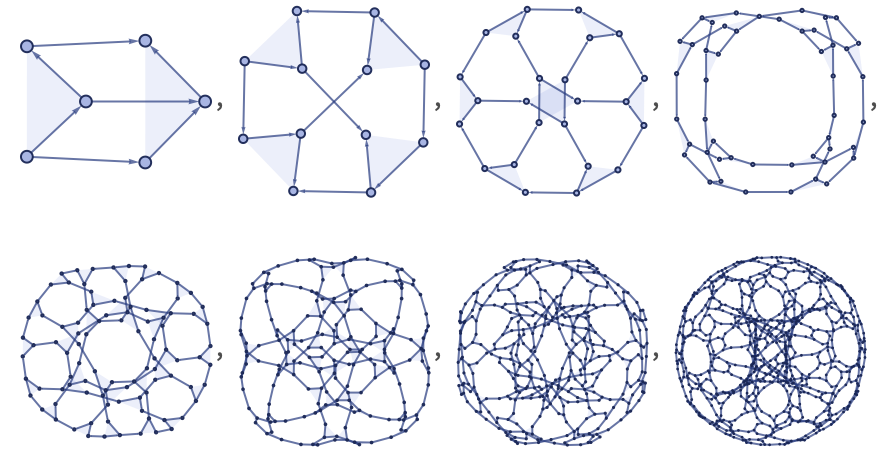
Visualized in 3D after 10 steps (and reconstructing less of the surface), this becomes:

  • Download Notebook
  • Open in Wolfram Cloud
  • Discuss This Page